Skip to main content
About course

SG2214 Fluid Mechanics 7.5 credits

Administer About course

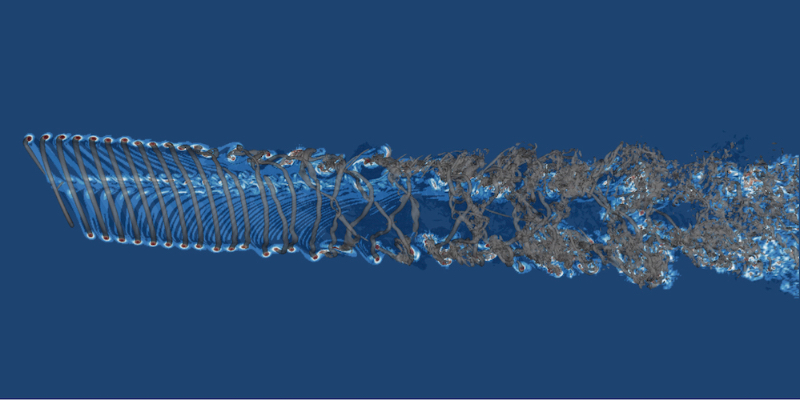
All fluid flows are governed by a single set of partial differential equations, the Navier-Stokes equations. This includes for instance, the aerodynamics of bumble bees and aerospace planes, the turbulence around vehicles and in the atmosphere and the convection in the sun and around a human body. The course is an in-depth introduction to fluid mechanics, with an emphasis of understanding fluid phenomena using on the Navier-Stokes equations. The equations are derived in detail and numerous examples of solutions are presented. Fluid Mechanics also has many important applications in engineering, geo- and astro-physics and bio-physics, for example, which makes this course ideal as a starting point for students with a varied interest in applications.

Choose semester and course offering

Choose semester and course offering to see current information and more about the course, such as course syllabus, study period, and application information.

Headings with content from the Course syllabus SG2214 (Spring 2022–) are denoted with an asterisk ( )

Content and learning outcomes

Course contents

Introduction, tensors, kinematics. Continuum mechanical conservation laws for mass, momentum and energy. Laminar viscous flow. Laminar boundary layers. Vorticity dynamics. Two-dimensional irrotational flow. Introduction to turbulent flow.

Intended learning outcomes

  1. The student should be able to identify, apply and/or present derivations of mathematical models of fluid mechanical phenomena and make relevant approximations.
  2. The student shall for simplified cases be able to apply the derived models (numerically or theoretically) and be able to interpret the result.
  3. The student should show an ability to relate obtained data, observed phenomena and processes in a laboratory environment to the theoretical description of fluid mechanics.
  4. The student should get a fundamental preparation in order to be able to work with fluid mechanical problems as an engineer.

Course disposition

No information inserted

Literature and preparations

Specific prerequisites

Completed BSc course in fluid mechanics and completed BSc thesis.

English B / English 6

Recommended prerequisites

The student should have good knowledge in linear algebra and calculus in more than one variable, vector analysis, Gauss and Stokes theorems and solution of elementary partial differential equations, basic knowledge of fluid mechanics phenomena, computer programming in e.g. Matlab.

Equipment

No information inserted

Literature

No information inserted

Examination and completion

If the course is discontinued, students may request to be examined during the following two academic years.

Grading scale

A, B, C, D, E, FX, F

Examination

  • INL1 - Assignments, 3.0 credits, grading scale: P, F
  • TEN1 - Examination, 4.5 credits, grading scale: A, B, C, D, E, FX, F

Based on recommendation from KTH’s coordinator for disabilities, the examiner will decide how to adapt an examination for students with documented disability.

The examiner may apply another examination format when re-examining individual students.

INL1: Written assignments and participation and reporting of laboratory exercise

TEN1: Written exam.

Examiner decides, in consultation with KTH's coordinator for students with disability (Funka), about any adapted examination for students with documented, permanent disability. The examiner may allow another examination form when re-examining individual students.

Other requirements for final grade

  • INL1, Assignments/laboratory exercise, 3,0 hp, P/F
  • TEN1, Examination, 4,5 hp, A-F

Opportunity to complete the requirements via supplementary examination

No information inserted

Opportunity to raise an approved grade via renewed examination

No information inserted

Examiner

Ethical approach

  • All members of a group are responsible for the group's work.
  • In any assessment, every student shall honestly disclose any help received and sources used.
  • In an oral assessment, every student shall be able to present and answer questions about the entire assignment and solution.

Further information

Course web

Further information about the course can be found on the Course web at the link below. Information on the Course web will later be moved to this site.

Course web SG2214

Offered by

Main field of study

Mechanical Engineering

Education cycle

Second cycle

Add-on studies

SG2218 Turbulence

SG2215 Compressible flow

SG2221 Wave motions and hydrodynamic stability

SG2212 Computational Fluid Mechanics

Contact

Anders Dahlkild (aad@kth.se), Shervin Bagheri (sherwinb@kth.se)

Supplementary information

The course is compulsory for students in Fluid Mechanics track in Masters program in Engineering Mechanics.